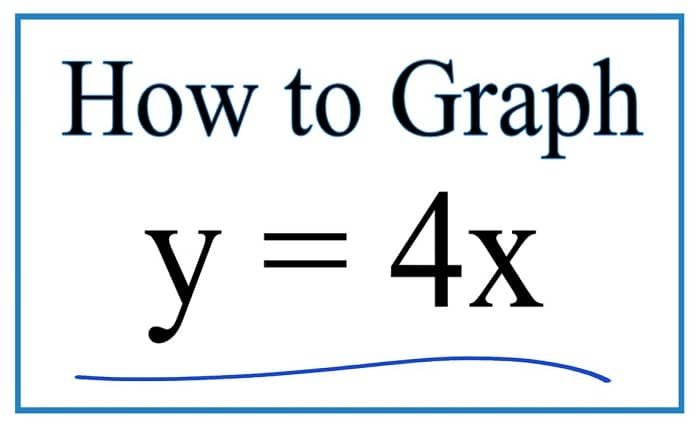
Introduction
Linear equations are equations between two variables that produce a straight line when graphed. The equation y = 4x is a classic example of a linear equation, representing a direct proportionality between the variables y and x.
Slope
In the equation y = 4x, the number 4 is known as the slope. The slope indicates how steep the line is. For every unit increase in x, y increases by 4 units. This makes y = 4x a line that rises sharply compared to equations with smaller slopes.
The Y-Intercept
The y-intercept is the point where the line crosses the y-axis. For the equation y = 4x, the y-intercept is 0. This means the line passes through the origin (0,0), indicating that when x is 0, y is also 0.
Graphing y = 4x
To graph y = 4x, you plot points where x and y correspond according to the equation. For example, if x is 1, y is 4; if x is 2, y is 8, and so on. Connecting these points with a straight line gives you the graph of y = 4x.
Solving for x and y
The equation y = 4x can be manipulated to solve for either variable. For example, if y is given, you can find x by dividing y by 4. Conversely, if x is given, you can find y by multiplying x by 4. This flexibility makes y = 4x a useful equation in various problems.
Applications in Real Life
The equation y = 4x is not just theoretical. It can model real-world situations where one quantity changes at a constant rate relative to another. For instance, if a car travels at 4 miles per hour, the distance traveled (y) after x hours can be described by y = 4x.
Importance in Mathematics
Understanding y = 4x is crucial for learning more advanced concepts in algebra and calculus. It introduces the idea of linearity, which is foundational for understanding more complex mathematical relationships.
Exploring Parallel and Perpendicular Lines
Lines described by equations like y = 4x can be compared to other lines to understand parallelism and perpendicularity. For example, any line with the equation y = 4x + b (where b is any constant) will be parallel to y = 4x. A line perpendicular to y = 4x would have a slope of -1/4.
Using Technology to Visualize y = 4x
Graphing calculators and computer software can help visualize the equation y = 4x. These tools allow you to see the relationship between x and y more clearly and can be helpful for students learning about linear equations.
Common Mistakes to Avoid
When working with y = 4x, it’s easy to make mistakes like mixing up the slope and y-intercept or plotting points incorrectly. Always double-check your calculations and understand the role of each component in the equation.
Conclusion
The equation y = 4x is a simple yet powerful tool in mathematics. Its straightforward nature makes it an excellent starting point for learning about linear relationships. Whether you’re graphing the equation, solving for variables, or applying it to real-world scenarios, y = 4x offers valuable insights.
FAQ
1.What does the equation y = 4x represent?
The equation y = 4x represents a linear relationship where y is four times the value of x.
2.How do you graph y = 4x?
To graph y = 4x, plot points where the coordinates (x, y) satisfy the equation and connect them with a straight line.
2.What is the slope of y = 4x?
The slope of y = 4x is 4, indicating a steep rise.
3.Can y = 4x be used in real-life situations?
Yes, y = 4x can model scenarios where one variable changes at a constant rate relative to another, such as speed and distance.
4.What is the y-intercept of y = 4x?
The y-intercept of y = 4x is 0, meaning the line passes through the origin (0,0).