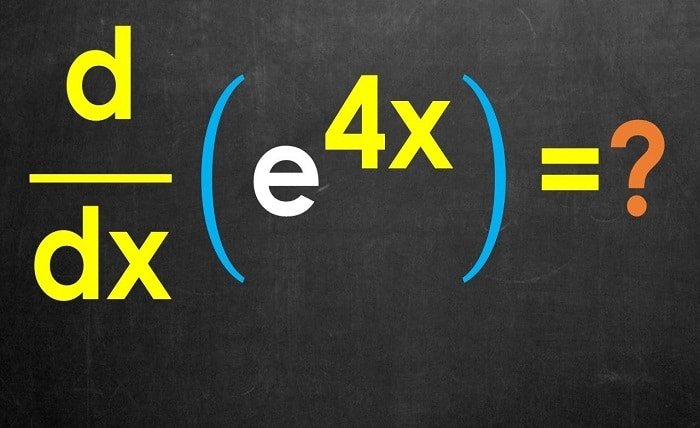
The derivative of a function is a fundamental concept in calculus, representing the rate at which the function’s value changes at a given point. For students and professionals alike, understanding how to calculate and interpret derivatives is crucial. This blog post delves into the derivative of the simple linear function 4x, a common example used in introductory calculus courses.
What is a Derivative?
The derivative of a function measures how the function’s output changes as its input changes. In the case of the derivative of 4x, it provides insights into the slope of the line represented by the equation y = 4x. Understanding this concept is crucial for tackling more complex calculus problems.
Calculating the Derivative of 4x
Calculating the derivative of 4x is straightforward. According to the power rule in calculus, the derivative of ax^n is nax^(n-1). Applying this rule, the derivative of 4x, where a = 4 and n = 1, is simply 4. This shows that the slope of the line y = 4x is constant, and the rate of change does not vary with x.
The Power Rule Explained
The power rule is a basic yet powerful tool in differential calculus. It allows for quick differentiation of any polynomial and is particularly useful when dealing with linear functions like 4x. By understanding this rule, students can handle more complex differentiation tasks efficiently.
Visualizing the Derivative of 4x
A visual approach can often clarify the concept of derivatives. Graphing the function y = 4x and its derivative helps illustrate that the slope of 4 is constant across the entire line. This graphical representation is an excellent way for visual learners to grasp the concept of derivatives.
Application in Real-World Problems
The derivative of 4x isn’t just a theoretical concept; it has practical applications in various fields such as physics, economics, and engineering. For instance, if 4x represents the speed of an object, then its derivative, being constant, indicates a steady velocity.
Derivative and Motion
Connecting derivatives to motion provides a tangible understanding of calculus. In the context of the derivative of 4x, if x represents time and y represents distance, the constant derivative implies constant speed, a fundamental concept in kinematics.
The Importance of the Constant Derivative
The constancy of the derivative of 4x teaches a valuable lesson in calculus: not all functions exhibit complex behaviors. Some, like linear functions, are predictable and serve as the building blocks for understanding more intricate functions.
Teaching Strategies for the Derivative of 4x
Educators can use the derivative of 4x as a stepping stone to introduce more complex calculus concepts. It serves as an excellent example for demonstrating basic differentiation rules and can be used to transition smoothly into non-linear functions.
Common Mistakes to Avoid
When studying the derivative of 4x, students may overlook the simplicity of the function. It’s important to recognize that while the derivative is constant, the underlying principles apply universally across different types of functions.
Advanced Concepts Related to Derivative of 4x
For those looking to extend their knowledge, exploring how the derivative of 4x applies to higher dimensions or in differential equations can be enlightening. This exploration can lead to a deeper understanding of calculus and its applications.
Conclusion
The derivative of 4x is a fundamental concept that serves as a cornerstone in the study of calculus. It exemplifies how simple rules can govern the behavior of functions and provides a clear example of constant rate change. This discussion not only aids in educational contexts but also enriches practical understanding in various scientific and engineering fields.
FAQs
1. What does the derivative of 4x tell us in practical terms?
The derivative of 4x tells us that the rate of change of the function y = 4x is constant, which in practical terms can represent constant speed or rate of growth.
2. Why is the derivative of 4x always 4?
The derivative of 4x is always 4 because the power rule in calculus states that the derivative of ax^n, where n is 1, is 1*ax^(1-1), which simplifies to a.
3. How can the derivative of 4x be applied in economics?
In economics, the derivative of 4x can represent a constant rate of increase in cost or revenue when x is the quantity of goods produced or sold.
4. Can the derivative of 4x change under different conditions
No, the derivative of 4x is a constant 4, as it is derived from a linear function with a constant slope.
5. How can visualizing the derivative help in understanding it better?
Visualizing the derivative through graphs shows that the slope of the tangent line to the curve y = 4x is always the same, reinforcing the concept of constant rate of change.





